Dispersive Qubit Readout with Intrinsic Resonator Reset

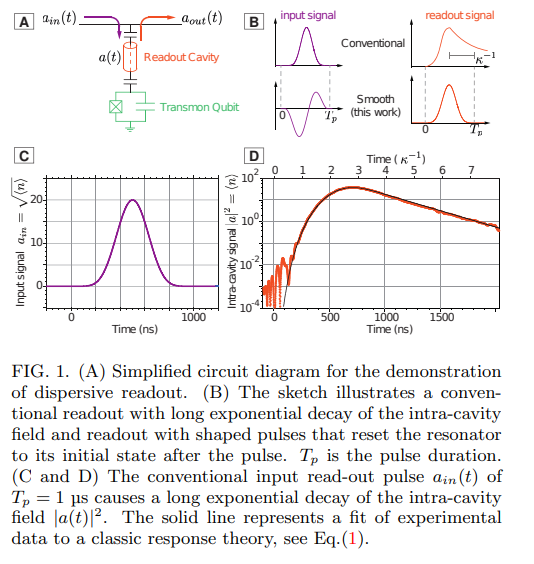
     A key challenge in quantum computing is speeding up measurement and initialization. Here, we experimentally demonstrate a dispersive measurement method for superconducting qubits that simultaneously measures the qubit and returns the readout resonator to its initial state. The approach is based on universal analytical pulses and requires knowledge of the qubit and resonator parameters, but needs no direct optimization of the pulse shape, even when accounting for the nonlinearity of the system. Moreover, the method generalizes to measuring an arbitrary number of modes and states. For the qubit readout, we can drive the resonator to ∼ 102 photons and back to ∼ 10−3 photons in less than 3κ −1 , while still achieving a T1-limited assignment error below 1%. We also present universal pulse shapes and experimental results for qutrit readout.